From the work of Stein-Watkins, an initial database was made of elliptic curves with discriminant D of absolute value less than or equal to 1012, with conductor either less than 108 or prime conductor less than 1010. However, this list was not verified to be complete, due to concerns such as minimal quadratic twists. The approach was to fix minimal c-invariants of the elliptic curve, modulo certain conditions, such that 1728D=c34-c26. c4 was chosen to be not more than 1.44 × 1012. However, this bound on the c4, as via the ABC Conjecture, did not necessarily guarantee that the list would be comprehensive, which presented us with our task.
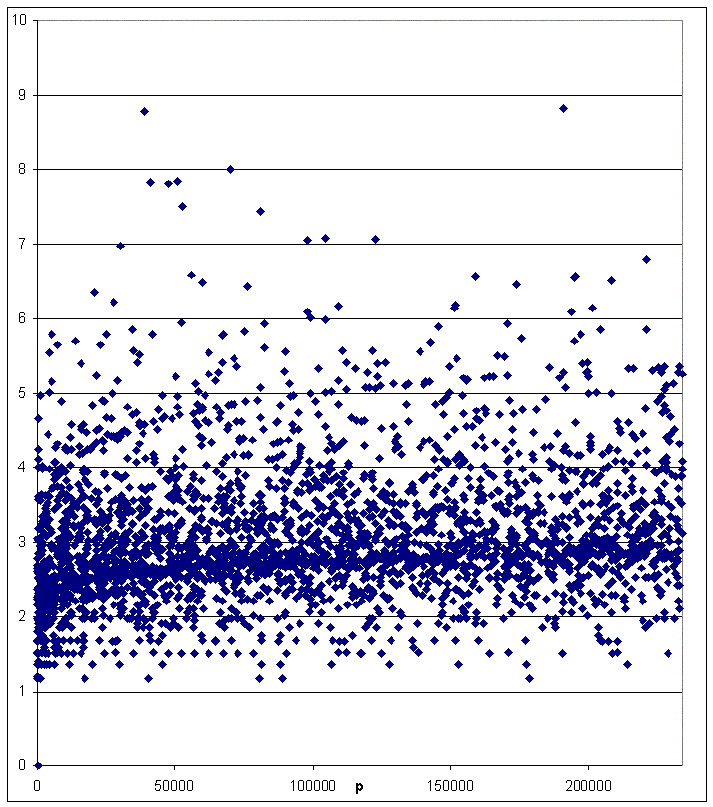
Below is a graph of the c4 invariants of all
elliptic curves of conductor p found by Stein-Watkins:

Our project stemmed from an implementation of Mestre's algorithm in MAGMA on the supercomputers Meccah and Neron. The final result of this investigation, then, whether new elliptic curves were to be found or not, would be a "proof by computer." The material presented henceforth are the results of these computations, and as such, assume that the computations ran without any errors due to software or hardware bugs.