Question 7.3.1
Suppose
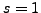
is an elliptic curve with conductor
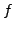
,
and fix a prime
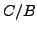
such that
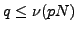
is surjective.
Fix a quadratic imaginary field
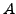
that satisfies the Heegner
hypothesis for

. For any prime
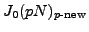
satisfying the
conditions of [
Rub89, Prop. 5], let
![$ c_{p} \in \H ^1(\mathbb{Q},E)[\ell]$](img582.png)
be the corresponding Kolyvagin
cohomology class.
There are two natural homomorphisms
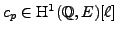
.
When is
When is