Discriminants of Hecke Algebras

Let $R$ be a ring and let $A$ be an $R$ algebra that is free as an $R$ module. The trace of an element of $A$ is the trace, in the sense of linear algebra, of left multiplication by that element on $A$.

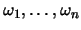
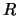
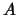
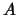
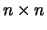
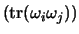
Definition 2.1 (Discriminant)   Let $\omega_1,\ldots,\omega_n$ is a $R$-basis for $A$. Then the discriminant of $A$, denoted $\disc(A)$, is the determinant of the $n\times n$ matrix $(\tr(\omega_i\omega_j))$, which is well defined modulo squares of units in $A$.

When $R=\mathbb{Z}$ the discriminant is well defined, since the only units are $\pm 1$.

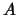
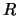
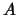
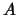
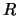
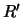
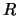
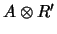
Proposition 2.2   Suppose $R$ is a field. Then $A$ has discriminant 0 if and only if $A$ is separable over $R$, i.e., for every extension $R'$ of $R$, the ring $A\otimes R'$ contains no nilpotents.

The following proof is summarized from Section 26 of Matsumura. If $A$ contains a nilpotent then that nilpotent is in the kernel of the trace pairing. If $A$ is separable then we may assume that $R$ is algebraically closed. Then $A$ is an Artinian reduced ring, hence isomorphic as a ring to a finite product of copies of $R$, since $R$ is algebraically closed. Thus the trace form on $A$ is nondegenerate.