Next: Finite Continued Fractions Up: Elementary Number Theory, A Previous: Exercises Contents Index
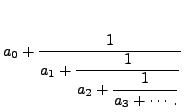
In this book we will assume that the ![]() are real numbers and
are real numbers and
![]() for
for ![]() , and the expression may or may not go on
indefinitely. More general notions of continued fractions have been
extensively studied, but they are beyond the scope of this book.
We will be most interested in the case when the
, and the expression may or may not go on
indefinitely. More general notions of continued fractions have been
extensively studied, but they are beyond the scope of this book.
We will be most interested in the case when the ![]() are all
integers.
are all
integers.
We denote the continued fraction displayed above by
For example,
![$\displaystyle [1,2] = 1+\frac{1}{2} = \frac{3}{2},$](img1552.png)
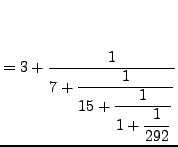 |
||
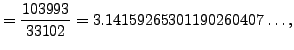 |
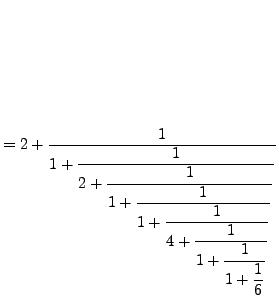 |
||
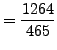 |
||
Continued fractions have many applications. For example, they
provide an algorithmic way to recognize a decimal
approximation to a rational number. Continued fractions also suggest
a sense in which ![]() might be ``less complicated'' than
might be ``less complicated'' than ![]() (see
Example 5.2.4 and Section 5.3).
(see
Example 5.2.4 and Section 5.3).
In Section 5.1 we study continued fractions
![]() of finite length and lay the foundations for
our later investigations. In Section 5.2 we give the
continued fraction procedure, which associates to a real number
of finite length and lay the foundations for
our later investigations. In Section 5.2 we give the
continued fraction procedure, which associates to a real number ![]() a
sequence
a
sequence
![]() of integers such that
of integers such that
![]() . We also prove that if
. We also prove that if
![]() is any
infinite sequence of positive integers, then the sequence
is any
infinite sequence of positive integers, then the sequence
![]() converges; more generally, we prove that if
the
converges; more generally, we prove that if
the ![]() are arbitrary positive real numbers and
are arbitrary positive real numbers and
![]() diverges then
diverges then ![]() converges. In Section 5.4, we
prove that a continued fraction with
converges. In Section 5.4, we
prove that a continued fraction with
![]() is (eventually)
periodic if and only if its value is a non-rational root of a
quadratic polynomial, then discuss open questions concerning continued
fractions of roots of irreducible polynomials of degree greater
than
is (eventually)
periodic if and only if its value is a non-rational root of a
quadratic polynomial, then discuss open questions concerning continued
fractions of roots of irreducible polynomials of degree greater
than ![]() . We conclude the chapter with applications of continued
fractions to recognizing approximations to rational numbers
(Section 5.5) and writing integers as sums
of two squares (Section 5.6).
. We conclude the chapter with applications of continued
fractions to recognizing approximations to rational numbers
(Section 5.5) and writing integers as sums
of two squares (Section 5.6).
The reader is encouraged to read more about continued fractions in [#!hardywright!#, Ch. X], [#!khintchine!#], [#!burton!#, §13.3], and [#!niven-zuckerman-montgomery!#, Ch. 7].