Next: The BSD Rank Conjecture Up: The BSD Rank Conjecture Previous: The BSD Rank Conjecture Contents Index
An excellent reference for this section is Andrew Wiles's Clay Math Institute paper [Wil00]. The reader is also strongly encouraged to look Birch's original paper [Bir71] to get a better sense of the excitement surrounding this conjecture, as exemplified in the following quote:
``I want to describe some computations undertaken by myself and Swinnerton-Dyer on EDSAC by which we have calculated the zeta-functions of certain elliptic curves. As a result of these computations we have found an analogue for an elliptic curve of the Tamagawa number of an algebraic group; and conjectures (due to ourselves, due to Tate, and due to others) have proliferated.''
An elliptic curve ![]() over a field
over a field ![]() is the projective closure
of the zero locus of a nonsingular affine curve
is the projective closure
of the zero locus of a nonsingular affine curve
An elliptic curve ![]() has genus
has genus ![]() , and the set of points on
, and the set of points on ![]() has
a natural structure of abelian group, with identity element the one
extra projective point at
has
a natural structure of abelian group, with identity element the one
extra projective point at ![]() . Again, there are simple algebraic
formulas that, given two points
. Again, there are simple algebraic
formulas that, given two points ![]() and
and ![]() on an elliptic curve,
produce a third point
on an elliptic curve,
produce a third point ![]() on the elliptic curve. Moreover,
if
on the elliptic curve. Moreover,
if ![]() and
and ![]() both have coordinates in
both have coordinates in ![]() , then so does
, then so does ![]() .
The Mordell-Weil group
.
The Mordell-Weil group
In the 1920s, Mordell proved that if ![]() , then
, then ![]() is finitely
generated, and soon after Weil proved that
is finitely
generated, and soon after Weil proved that ![]() is finitely
generated for any number field
is finitely
generated for any number field ![]() , so
, so
Fix an elliptic curve ![]() over
over ![]() . For all but finitely
many prime numbers
. For all but finitely
many prime numbers ![]() , the equation (1.1.1)
reduces modulo
, the equation (1.1.1)
reduces modulo ![]() to define an elliptic curve
over the finite field
to define an elliptic curve
over the finite field ![]() .
The primes that must be excluded are exactly the primes that divide
the discriminant
.
The primes that must be excluded are exactly the primes that divide
the discriminant ![]() of (1.1.1).
of (1.1.1).
As above, the set
of points
![]() is an abelian group. This group
is finite, because it is contained in the set
is an abelian group. This group
is finite, because it is contained in the set
![]() of
rational points in the projective plane. Moreover,
since it is the set of points on a (genus 1) curve,
a theorem of Hasse implies that
of
rational points in the projective plane. Moreover,
since it is the set of points on a (genus 1) curve,
a theorem of Hasse implies that
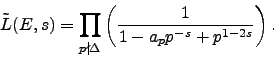
A deep theorem of Wiles et al. [Wil95,BCDT01], which many consider the crowning
achievement of 1990s number theory, implies that
![]() can be analytically continued to an analytic function
on all
can be analytically continued to an analytic function
on all ![]() . This implies that
. This implies that
![]() has a Taylor series
expansion about
has a Taylor series
expansion about ![]() :
:
This problem is extremely difficult. The conjecture was made in the 1960s, and hundreds of people have thought about it for over 4 decades. The work of Wiles et al. on modularity in late 1999, combined with earlier work of Gross, Zagier, and Kolyvagin, and many others proves the following partial result toward the conjecture.
In 2000, Conjecture 1.1 was declared a million dollar
millenium prize problem by the Clay Mathematics Institute, which
motivated even more work, conferences, etc., on the conjecture. Since
then, to the best of my knowledge, not a single new result directly
about Conjecture 1.1 has been
proved![]() .
The class of curves for which
we know the conjecture is still the set of curves over
.
The class of curves for which
we know the conjecture is still the set of curves over ![]() with
with
![]() , along with a finite set of individual curves on
which further computer calculations have been performed (by Cremona,
Watkins, myself, and others).
, along with a finite set of individual curves on
which further computer calculations have been performed (by Cremona,
Watkins, myself, and others).
``A new idea is needed.''
- Nick Katz on BSD, at a 2001 Arizona Winter School
And another quote from Bertolini-Darmon (2001):
``The following question stands as the ultimate challenge concerning the Birch and Swinnerton-Dyer conjecture for elliptic curves over: Provide evidence for the Birch and Swinnerton-Dyer conjecture in cases where
.''
William 2007-05-25